Probability bounds for M-Skorohod oscillations

Florin Avram
Murad S. Taqqu

Abstract:

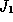Billingsley developed a widely used method for proving weak convergence with respect to the sup-norm and -Skorohod topologies, once convergence of the finite-dimensional distributions has been established. Here we show that Billingsley's method works not only for J oscillations, but also for M oscillations. This is done by identifying a common property of the J and M functions, called sub-triadditivity, and then showing that Billingsley's approach in the case of the J function can be adequately modified to apply to any sub-triadditive function.