How do conditional moments of stable vectors depend on the spectral measure?

Renata Cioczek-Georges
Murad S. Taqqu

Abstract:

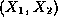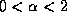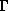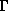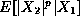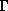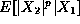Let be an -stable random vector with , not necessarily symmetric. Its distribution is characterized by a finite measure on the unit circle called the spectral measure. It is known that if satisfies some integrability condition then the conditional moment can exist for some values of p greater than . This paper provides a sufficient condition on for the existence of the conditional moment involving the maximal range of possible p's, namely 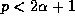.